A steady progression of motor dysfunction takes place in Huntington’s disease¹ (HD). The origin of this disturbance with relation to the motor control process is not understood. Here we studied reaching movements in asymptomatic HD gene-carriers (AGCs) and subjects with manifest HD. We found that movement jerkiness, which characterizes the smoothness and efficiency of motion, was a sensitive indicator of presymptomatic HD progression. A large fraction of AGCs displayed elevated jerk even when more than 7 years remained until predicted disease onset. Movement termination was disturbed much more than initiation yet was highly variable from trial to trial. Analysis of this variability revealed that the sensitivity of end-movement jerk to subtle, self-generated early-movement errors was greater in HD subjects than in controls. Additionally, we found that HD corrective responses to externally-generated force pulses were dramatically disturbed, indicating that HD subjects display aberrant responses to both external and self-generated errors. Because feedback corrections are driven by error and are delayed such that they predominantly effect movement termination, these findings suggest that a dysfunction in error correction characterizes the motor control deficit in early HD. This dysfunction may be observed years before clinical disease onset and it worsens with disease progression.

Huntington’s disease is an autosomal dominant inherited neurologic disorder caused by a glutamate repeat expansion in the IT15 gene². Disease symptoms appear in the fourth or fifth decade of life¹, and then progress steadily during the succeeding 10-20 years. Significant, progressive atrophy of the basal ganglia is evident in HD³, and may even be detectable before clinical onset⁴. It’s hallmark motor sign is chorea: the occurrence of rapid, irregular, and arrhythmic complex involuntary movements. Along with chorea, substantial impairment of voluntary movement also occurs¹, and it may be of greater functional importance in the lives of patients⁵. Two control processes take part in the execution of arm movements. Feedforward control is the generation of motor commands based a priori on the desired action and an internal model of the system’s response^6,7, while feedback control entails the ‘mid-flight’ correction of these commands based on errors detected during their execution⁸. To study these processes in HD, we used a high performance manipulandum⁹ to record visually guided reaching arm movements in patients with HD and presymptomatic individuals with the HD mutation up to 18 years from predicted disease onset.

Figure 1 displays hand paths of the two most regular and two least regular movements in each direction, for several subjects after 200 practice movements. The range of movement quality is generally much larger in HD patients than controls. Some movements made by symptomatic HD subjects appear normal while others are markedly irregular. Specifically, many HD movements have large changes in direction, smooth or abrupt, when approaching the target, while almost all movements fail to stop efficiently and smoothly. One way to characterize these irregularities is to quantify the smoothness of each movement. Smoothness can be defined as the lack of abrupt change, so a trajectory that minimizes abrupt changes in a variable will maximize its smoothness. Human reaching movements are of near maximal acceleration smoothness, quantified by minimal cumulative squared acceleration change¹⁰, also referred to as minimum total squared jerk.

Open in a separate window

Figure 1

Hand paths from selected subjects after 200 practice trials (movements 201-300). a, The two most regular movements in each direction. b, The two least regular movements each direction. Movement regularity was determined by the correlation coefficient⁶ of the velocity profile with the velocity profile of that subject’s typical movement. The typical movement was defined as the movement in each direction with the highest average correlation to other movements. Hand paths are plotted from the center out relative to their starting positions. Points are spaced 30ms apart in time. The distance between consecutive points is proportional to the movement speed during that interval. Top row: subjects with manifest HD. Second row: asymptomatic gene-carriers. Third row: controls who have a parent with HD but who are mutation negative. Bottom row: controls age matched to the asymptomatic gene-carriers. The letter that labels each subject identifies him or her in figure 2.

Figure 2a shows that all HD patients and several AGCs made movements with above normal jerk. Notably, all HD subjects reduced their movement jerk with practice, although not nearly to control levels. The smoothness of movements increased over time even in subjects who were markedly more jerky than normal. In order to compare the initiation and completion of movement, we split each movement at the peak in the speed profile. This is roughly the point at which movement toward the target switches from acceleration to deceleration. Comparison of the total squared jerk before and after the peak in the speed profile (figure 2b) reveals that while fewer than half of the HD patients have high jerk during both parts of the movement, all have above normal post-peak jerk. Only 2 of 16 AGCs show above normal pre-peak jerk, but a majority have high post-peak jerk. To assess disease progression in AGCs, we used an estimate of disease onset age based on each subject’s parental onset age and glutamate repeat length⁴. The amount of post-peak jerk correlated significantly (r=-0.62) with estimated time to disease onset for AGCs while the pre-peak jerk did not (r=0.02). Post-peak jerk was above normal in 4 of 5 close to onset (<7 years) subjects and in 3 of 9 far from onset subjects. As groups, both HD patients and AGCs had significantly higher than normal post-peak jerk (p<10^-6, p<0.00012) (see figure 2e). Moreover, AGCs who were close to predicted disease onset (< 7 years) had significantly higher jerk in the third set than far from onset (> 7 years) subjects (p<0.0062) who in turn had significantly higher jerk than controls (p<0.013).

Open in a separate window

Figure 2

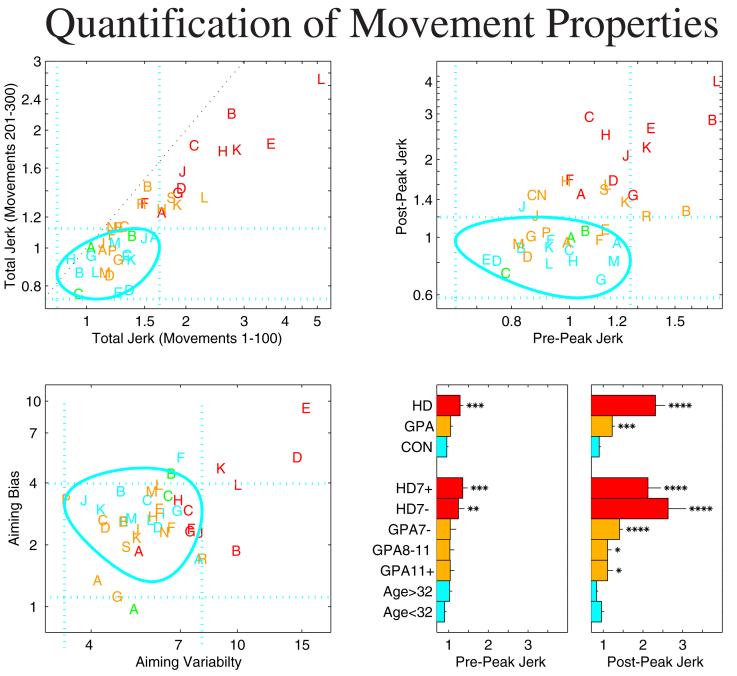
Quantification of movement properties. Red: subjects with manifest HD. Orange: asymptomatic gene-carriers. Blue: age matched controls. Green: gene-negative individuals having a parent with HD. Note that a-c are plotted on a logarithmic scale for clarity. The blue dotted lines show the 95% confidence intervals for the control distribution (mean +/- 1.96 standard deviations). The black diagonal line in a represents the axis of equality (y=x). a, The mean of the normalized total squared jerk for all subjects in the first 100 movements and in movements 201-300. All symptomatic subjects and a subset of asymptomatic gene-carriers have higher than normal jerk. b, The mean of normalized jerk (in movements 201-300) before and after the peak in the movement speed. The pre-peak segment, which reflects movement initiation, appears much less disturbed in HD than post-peak segment, which reflects movement completion. c, Aiming bias and aiming variability. We refer to aiming as the direction of travel with respect to the initial target direction, during the pre-peak movement segment. Like pre-peak jerk, aiming reflects the quality of movement. d,e, Group-wise comparisons of normalized pre-peak and post-peak jerk, respectively. Asterisks indicate significantly worse performance than control subjects. * p<0.05. ** p<0.01. *** p<0.001. **** p<0.0001. HD7+: Subjects with manifest HD for more than 7 years. HD7-: Subjects with manifest HD for less than 7 years. GPA7-: Gene-carriers less than 7 years from predicted onset. GPA7-11: Gene-carriers 7 to 11 years from predicted onset. GPA11+: Gene-carriers more than 11 years from predicted onset. Age>32: controls more than 32 years old. Age<32: controls less than 32 years old.

Figure 2c shows that initial aiming is not dramatically disturbed in HD. Average directional aiming bias (see methods) is normal in 9 of 11 HD patients. Aiming variability is in the control range for a majority (6 of 11) of patients, although as a group, subjects with manifest HD display increased aiming variability. All AGCs have normal aiming biases of 3-7 degrees and none has above normal aiming variability.

These results suggest that HD movements often begin normally, but become jerky and irregular at some point during their course. Comparison of the average time course of raw squared jerk profiles between groups for movements in different speed ranges (figure 3), reveals a strikingly consistent pattern. At each speed range, the HD jerk profile closely matches the control profile during the beginning of movement, but begins to separate from it 200-300 msec after onset. Note that the end movement squared jerk is 10-30 times greater in HDs than controls.

Open in a separate window

Figure 3

Squared jerk profiles for different movement speeds. Average raw squared jerk ± standard error is plotted on a logarithmic scale as function of time since movement onset for movements in each speed range, in different subject groups. For reference, the second peak in the squared jerk profile approximately corresponds to the peak in the speed profile. Note that at all speed ranges the separate groups have quite similar jerk profiles until 300ms, but HD subjects have very different profiles after this point.

Why might HD movements begin to become irregular 200-300ms into their course and not before? Corrective actions based on visual^11,12 and proprioceptive¹³ information acquired during reaching movements begin to take place at about the time at which HD movements become irregular, so one possibility is that the system that generates these corrective actions is disturbed. Alternatively, there may be a disturbance in decelerating movement or another end-movement process independent of error correction.

To distinguish between these possiblities, we characterized the performance of the error feedback control system in HD. If the system that generates corrective actions during movement (the feedback controller) is affected in HD, then a strong relationship should exist between the magnitude of error early in the movement and size of disturbance later. Error, the difference between desired system state and actual state, is the primary input to a feedback controller. If the error during a movement is small, then large corrective actions are not necessary and dysfunction in feedback control might have only minimal effects. However, if errors are large, substantial corrective actions are required, and dysfunctional feedback control may cause significant problems with movement.

While we can measure actual movements quite accurately, we have no reliable way of estimating the desired motion state within a single movement. This makes direct estimate of error infeasible. If errors are symmetrically distributed, then the average movement profile should approximate the average motion plan. However, subjects may plan to move a bit faster on one trial then slower on the next, and these variations in the plan may be as large as the typical errors between plan and action. Although the motor plan might change across trials, a few of its properties are invariant. Velocity profiles of reaching movements are symmetric and unimodal: the peak in the velocity profile occurs very close to the movement’s midway point, regardless of amplitude, direction, or speed¹⁴. Therefore, the distance between the midway point and the position where the peak speed occurs can be used as an indicator of error early in the movement.

The relationship between error early in movement and jerk late in movement is shown in figure 4. The probability distributions of peak speed positions (PSPs) do not differ dramatically between groups (figure 4a,c,e,g,i). This implies that amount of error early in the movement is comparable between groups. However, the reaction to this error is very different between groups. Figure 4b,d,f,h,j show that the jerk that occurs after the hand reaches the PSP varies considerably with PSP in all subject groups. When the PSP is far from the midway point, indicating large error early in the movement, post-peak jerk is high. However, the sensitivity of post-peak jerk to PSP is much greater in HD patients and AGCs than controls. Figure 4h shows that the difference in mean post-peak jerk between controls and AGCs is much greater at large PSP displacements than at small displacements. Subjects with manifest HD (p=0.006) and AGCs (p=0.04) display significantly higher sensitivity in their response of end-movement jerk to early movement error than do controls, as measured by slope in this relationship. The sensitivity of post-peak jerk to PSP displacement indicates the degree to which end-movement smoothness depends on early-movement error. The increase in this sensitivity in HD subjects with and without clinically manifest symptoms suggests that an error dependent control process is disturbed early in the disease course and further deteriorates with disease progression.

Open in a separate window

Figure 4

Errors that occur early in the movement, before the hand reaches its peak speed, predict jerk that occurs later. a,c,e, The two-dimensional probability densities of peak speed positions (PSPs), for each group during movements 301-400. This is the likelihood of the peak speed occurring at each position. The x-axes are in the target direction, and the y-axes are in the direction perpendicular to the target. PSPs are clustered near the movement midway point. g, Summary of a,c,e: the average probability density at a given displacement between the PSP and movement midway point (50%, 0%). Large values for the distance from midway point to peak speed position indicate large early-movement errors, which are uncommon. The similarity of these distributions indicates that there are not large differences in the pattern of error recorded from the three subject groups early in the movement. b,d,f, End-movement jerk as a function of peak speed position. When large early movement errors occur, as indicated by large PSP displacements (near the image boundaries), the post-peak jerk is increased for all groups, but the increase is greater for asymptomatic and symptomatic HD subjects than controls. White color on images indicates no data. h, Summary of b,d,f: the average end-movement jerk at a given displacement between the PSP and movement midway point. i, average PSP displacement for each group. j, sensitivity of end movement jerk to PSP, measured as the slope of the relationship between them. Asterisks indicate significantly worse performance than control subjects. * p<0.05. ** p<0.01. *** p<0.001.

Previous reports have shown that cortical sensorimotor pathways are affected in patients with manifest HD. While short-loop reflexes that are mediated by spinal mechanisms are normal, long-loop reflexes, which involve the transfer of proprioceptive information through cortical pathways, are reduced or absent in HD^15,16. Cortical responses to peripheral nerve stimulation, as measured by somatosensory evoked potentials (SEPs), are also reduced in HD^15,17,18, suggesting that diminished cortical sensory input is responsible for the long-loop reflex reduction¹⁶. Additionally, a strong correlation exists between SEP deterioration and striatal glucose metabolism early in the course of HD¹⁷, hinting at a possible involvement of the striatum in the pathology of the SEPs and long-loop reflexes. Since cortical sensorimotor pathways play a large role in mediating error correction during voluntary movement, disruption of these pathways could lead to dysfunctional feedback control during movement.

Our analysis of the response to internal, self-generated errors suggested that error correction in general might be disturbed in HD. To test this hypothesis we externally imposed errors upon movements of these subjects via an occasional force pulse (70ms) shortly after movement initiation. These pulses were given randomly on a minority of trials, and could be in 8 different directions and of 3 different magnitudes: 6, 12, and 18 Newtons peak force. In addition to subjects with HD, we studied the performance of individuals with cerebellar deficits on this task. Hand paths of two symptomatic HD, one AGC, one control, and three cerebellar subjects for movements perturbed with 12 and 18 Newton force pulses are shown in figure 5a. These perturbations are substantial: trajectories can be altered by up to 5 cm (movement distance is 10cm). Control subjects correct the perturbations smoothly and efficiently, but extremely large successive overshoots are seen during certain HD movements. Not all HD movement corrections appear irregular, but some are so dramatically disturbed that they bear little qualitative similarity to any movements that we recorded from controls.

Several movements made by cerebellar subjects appear to have irregular and inefficient corrections, but close inspection of many of these trajectories reveals a striking similarity between the pattern of overshoots in these movements and the overshoots present in unperturbed movements in the same direction. This suggests that the irregularity that exists in these perturbed movements has a stronger relationship to the direction of movement than to the presence of perturbation. Note the movements toward 3:00, 4:30, and 7:30 for subjects 5 and 7.

HD subjects appear to have dysfunctional reactions to movement errors caused by external perturbations. During the post perturbation movement segment (the corrective period), jerk and path length are relatively increased over unperturbed movements significantly more for HD subjects than controls (see figure 5b) for both 12 and 18 N perturbations. The error-correction performance of AGCs generally falls between that of controls and subjects with manifest HD. Cerebellar subjects, like symptomatic HDs, had worse unperturbed movement performance than controls, but the decrement in their performance when perturbations were given was generally more like that of controls than HD subjects. This suggests that subjects with HD generally have greater deficits in error feedback control than do cerebellar patients.

The existence of performance deficits on tests of behavioral function before the clinical onset of HD is controversial. To date, assessments of motor, cognitive, or psychiatric function in HD have revealed only subtle deficits in presymptomatic subject groups^19,20,21 if any^22,23. When changes have been detected, they were not sufficiently specific to permit discrimination between mutation positive and mutation negative individuals, or even reliable identification of people with early stage manifest HD. In contrast, low glucose metabolism in the basal ganglia has been reported in about two thirds of asymptomatic at risk individuals tested^17,24,25 and basal ganglia volumes may be reduced years before clinical onset⁴, suggesting that brain pathology precedes manifestation of the behavioral dysfunction previously studied. However, end-movement jerk appears to be a sensitive indicator of HD progression, suggesting the existence of a direct behavioral correlate to the early brain pathology of HD.

Our results, which suggest relatively spared feedforward control but dysfunctional feedback control in HD, may help explain the pattern of motor learning deficits reported. Learning on rotary pursuit tasks, which involve long continuous movements largely under closed-loop feedback control, is impaired in HD^26,27. In contrast, learning to mirror trace polygons, which involves multiple short, discrete movements that rely more heavily on open-loop feedforward control, is intact in HD.

Real time error feedback control presents a formidable challenge to the CNS because of the large sensorimotor delays that occur²⁸. One way to improve the performance of such a time-delayed system is to include a component which can effectively predict away the delay and allow efficient error correction. This component could combine delayed sensory information with a knowledge of system’s physical dynamics and recent motor output to predict future motion state. This sort of predictor has been referred to as a forward model of system dynamics^8,29. Neurons in the basal ganglia have been shown to predict reward³⁰, hinting that predictive capacity may be a general feature of some basal ganglia structures. The main output of the basal ganglia modulates the action of the thalamus, which relays sensory information to the cortex. This information stream is likely to participate in error feedback control, thus the error correction process may be modulated by the basal ganglia, although the mechanism through which this occurs, including whether predictive capacity in basal ganglia structures is involved, is not yet understood.