Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-16T05:27:45.150Z Has data issue: false hasContentIssue false
  • English
  • Français

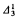-stability

Published online by Cambridge University Press:  12 March 2014

Dror Ben-Arié  and
Haim Judah
Dror Ben-Arié
Affiliation:
Department of Mathematics, Bar-Ilan University, Ramat-Gan 52900, Israel, E-mail: benarie@bimacs.cs.biu.ac.il
Haim Judah
Affiliation:
Department of Mathematics, Bar-Ilan University, Ramat-Gan 52900, Israel, E-mail: judah@bimacs.cs.biu.ac.il
Get access Rights & Permissions [Opens in a new window]

Abstract

We investigate the connection between -stability for random and Cohen forcing notions and the measurability and categoricity of the -sets. We show that Shelah's model for -measurability and categoricity satisfies -random-stability while it does not satisfy -Cohen-stability. This gives an example of measure-category asymmetry. We also present a result concerning finite support iterations of Suslin forcing.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 58 , Issue 3 , September 1993 , pp. 941 - 954
Copyright
Copyright © Association for Symbolic Logic 1993

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[BagJu]Bagaria, J. and Judah, H., Amoeba forcing, Souslin absoluteness and additivity of measure, Theory of the Continuum, (Judah, H., Just, , and Woodin, , editors), Springer-Verlag, New York, 1992.Google Scholar
[Bar]Bartoszyński, T., Additivity of measure implies additivity of category, Transactions of the American Mathematical Society, vol. 281 (1984), pp. 209–213.CrossRefGoogle Scholar
[BarJu]Bartoszyński, T. and Judah, H., Advanced set theory, K. Peters Publishers (to appear).Google Scholar
[Je]Jech, T., Set theory, Academic Press, New York, 1978.Google Scholar
[Jul]Judah, H., Absoluteness for projective sets, Lecture Notes in Mathematics, Springer-Verlag, Berlin (to appear).CrossRefGoogle Scholar
[Ju2]Judah, H., Exact equiconsistency results for sets of reals, Archive for Mathematical Logic,(to appear).Google Scholar
[Ju3]Judah, H., -sets: Measure and category, Israel Mathematical Conference Proceedings, vol. 6 (1993), pp. 361–384.Google Scholar
[JS1]Judah, H. and Shelah, S., Martin's axiom, measurability and equiconsistency results, this Journal, vol. 54 (1989), pp. 78–94.Google Scholar
[JS2]Judah, H. and Shelah, S., MA(σ-centered): Cohen reals, strong measure zero sets and strongly meager sets, Israel Journal of Mathematics, vol. 68 (1989), pp. 1–17.CrossRefGoogle Scholar
[JS3]Judah, H. and Shelah, S., Souslin forcing, this Journal, vol. 53 (1988), pp. 1188–1207.Google Scholar
[JS4]Judah, H. and Shelah, S., -sets of reals, Annals of Pure and Applied Logic, vol. 42 (1989), pp. 207–233.Google Scholar
[Ku]Kunen, K., Set theory—an introduction to independence proofs, North-Holland, Amsterdam, 1980.Google Scholar
[Pa]Pawlikowski, J., Why Solovay real produces Cohen real, this Journal, vol. 51 (1986), pp. 957–968.Google Scholar
[Sh]Shelah, S., Can you take Solovay's inaccessible away?, Israel Journal of Mathematics, vol. 48 (1984), pp. 1–47.CrossRefGoogle Scholar
[Tr]Truss, J., Sets having calibre ℵ1, Logic Colloquium 76 North-Holland, Amsterdam, 1977.Google Scholar