Europe PMC

This website requires cookies, and the limited processing of your personal data in order to function. By using the site you are agreeing to this as outlined in our privacy notice and cookie policy.

Metal Complexation for the Rational Design of Gemcitabine Formulations in Cancer Therapy.

Author information

Affiliations

  • 1. Department of Pharmaceutics and Center for Pharmaceutical Engineering and Sciences - School of Pharmacy, Virginia Commonwealth University, Richmond, Virginia 23284, United States.
      Authors
    • Carnamucio F 1
    • da Rocha SRP 1
    (2 authors)
  • 2. Dipartimento di Scienze Chimiche, Biologiche, Farmaceutiche ed Ambientali, Università di Messina, Viale F. Stagno d'Alcontres 31, 98166 Messina, Italy.
      Authors
    • Foti C 2
    • Cordaro M 2
    • Giuffrè O 2
    (3 authors)
  • 3. Institute for Chemical-Physical Processes National Research Council of Italy, Viale Ferdinando Stagno d'Alcontres, 37, 98158 Messina, Italy.
      Authors
    • Saija F 3
    • Cassone G 3
    (2 authors)

ORCIDs linked to this article

ACS Applied Materials & Interfaces, 08 Oct 2024, 16(42):56789-56800
https://doi.org/10.1021/acsami.4c12550 PMID: 39378358 PMCID: PMC11503523

This article is in the Europe PMC Open access subset. Refer to the copyright information in the article for licensing details.
Free full text in Europe PMC

Abstract 

Nanoformulation of chemotherapies represents a promising strategy to enhance outcomes in cancer therapy. Gemcitabine is a chemotherapeutic agent approved by the Food and Drug Administration for the treatment of various solid tumors. Nevertheless, its therapeutic effectiveness is constrained by its poor metabolic stability and pharmacokinetic profile. Nanoformulations of gemcitabine in lipid and polymer nanocarriers usually lead to poor loading capability and an inability to effectively control its release profile due to the physicochemical characteristics of the drug and matrices. Here, we propose metal-gemcitabine complexation with biorelevant metal cations as a strategy to alter the properties of gemcitabine in a noncovalent manner, paving the way for the development of novel nanoformulations. A speciation study on gemcitabine and Mn2+, Zn2+, and Ca2+ was performed with the aim of investigating the extent of the interaction between the drug and the proposed metal cations, and selecting the best conditions of temperature, pH, and drug-to-metal molar ratio that optimize such interactions. Also, a series of density functional theory calculations and spin-polarized ab initio molecular dynamics simulations were carried out to achieve insights on the atomistic modalities of these interactions. Mn2+-gemcitabine species demonstrated the ability to maintain gemcitabine's biological activity in vitro. The scientific relevance of this study lies in its potential to propose metal-gemcitabine as a valuable strategy for developing nanoformulations with optimized quality target product profiles. The work is also clinically relevant because it will lead to improved treatment outcomes, including enhanced efficacy and pharmacokinetics, decreased toxicity, and new clinical possibilities for this potent therapeutic molecule.

Free full text 

Logo of acssdLink to Publisher's site
ACS Applied Materials & Interfaces
ACS Appl Mater Interfaces. 2024 Oct 23; 16(42): 56789–56800.
Published online 2024 Oct 8. https://doi.org/10.1021/acsami.4c12550
PMCID: PMC11503523
PMID: 39378358

Metal Complexation for the Rational Design of Gemcitabine Formulations in Cancer Therapy

Federica Carnamucio,*† Claudia Foti,‡ Massimiliano Cordaro,‡ Franz Saija,§ Giuseppe Cassone,*§ Sandro R. P. da Rocha,† and Ottavia Giuffrè*||
Author information Article notes Copyright and License information Disclaimer

Associated Data

Supplementary Materials

Abstract

An external file that holds a picture, illustration, etc. Object name is am4c12550_0009.jpg

Nanoformulation of chemotherapies represents a promising strategy to enhance outcomes in cancer therapy. Gemcitabine is a chemotherapeutic agent approved by the Food and Drug Administration for the treatment of various solid tumors. Nevertheless, its therapeutic effectiveness is constrained by its poor metabolic stability and pharmacokinetic profile. Nanoformulations of gemcitabine in lipid and polymer nanocarriers usually lead to poor loading capability and an inability to effectively control its release profile due to the physicochemical characteristics of the drug and matrices. Here, we propose metal–gemcitabine complexation with biorelevant metal cations as a strategy to alter the properties of gemcitabine in a noncovalent manner, paving the way for the development of novel nanoformulations. A speciation study on gemcitabine and Mn2+, Zn2+, and Ca2+ was performed with the aim of investigating the extent of the interaction between the drug and the proposed metal cations, and selecting the best conditions of temperature, pH, and drug-to-metal molar ratio that optimize such interactions. Also, a series of density functional theory calculations and spin-polarized ab initio molecular dynamics simulations were carried out to achieve insights on the atomistic modalities of these interactions. Mn2+-gemcitabine species demonstrated the ability to maintain gemcitabine’s biological activity in vitro. The scientific relevance of this study lies in its potential to propose metal-gemcitabine as a valuable strategy for developing nanoformulations with optimized quality target product profiles. The work is also clinically relevant because it will lead to improved treatment outcomes, including enhanced efficacy and pharmacokinetics, decreased toxicity, and new clinical possibilities for this potent therapeutic molecule.

Keywords: gemcitabine, Mn2+, Zn2+, Ca2+, metal complexation, DFT calculation, ab initio, cancer therapy

Introduction

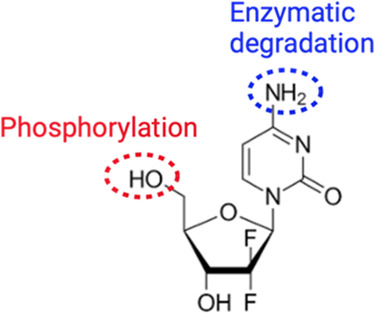
Gemcitabine or 2′-deoxy-2′,2′-difluorocytidine (GMT), whose structure is reported in Figure ​Figure11, is a cytidine analogue in which two fluorine atoms replace the hydroxyl on the ribose. GMT is intracellularly phosphorylated and converted to the active moiety dFdCDP and dFdCTP, which induce apoptosis by DNA recognition. After intravenous administration, ≥99% of GMT is rapidly deaminated to 2′,2′-difluorodeoxyuridine (dFdU) by deoxycytidine deaminase present in the blood and liver and then subjected to a fast renal clearance. GMT is approved by the Food and Drug Administration (FDA) for the treatment of various solid tumors, including pancreatic, breast, bladder, head, neck, thyroid, ovarian, nonsmall cell lung, bone, and biliary tract cancers.1,2 It is an essential medicine according to the World Health Organization (WHO).3 Administered as a prodrug, GMT is phosphorylated to form the active moiety GMT triphosphate (dFdCTP) and GMT diphosphate (dFdCDP), which inhibit DNA synthesis.4 Upon intravenous administration, it is rapidly deaminated by cytidine deaminase, and cleared by the kidneys.5 Over 99% of GMT is fully deaminated in the blood, liver, kidneys, and other tissues in the inactive metabolite 2′,2′-difluorodeoxyuridine (dFdU) and excreted by urine.6 While of tremendous benefit in the treatment of various types of malignancies, GMT has shown a rapid metabolism and a poor pharmacokinetic (PK) profile (plasma half-life T1/2 less than 30 min), thus limiting its potential as a chemotherapeutic agent.7 Due to its poor PK profile,7 high doses and prolonged infusion times are typically required to keep the concentrations within the therapeutic window, which may lead to myelosuppressive and other side effects.8,9 Nanodrug delivery systems have been proposed to improve GMT PK and biodistribution profiles, as they protect the drug against enzymatic degradation and, due to a GMT controlled release, improve PK tumor distribution.10

An external file that holds a picture, illustration, etc. Object name is am4c12550_0001.jpg

Gemcitabine (GMT) structure.

The use of nanoformulations in chemotherapies has been shown to be effective in decreasing toxicity, increasing selectivity and enhancing drug efficacy.11−13 Polymeric and lipid (e.g., liposomes) nanoparticles represent two prominent approaches in nanodrug delivery systems, particularly for anticancer drugs.14 Each approach offers distinct advantages. Liposomes, for instance, are highly biocompatible and biodegradable,15 whereas polymeric nanoparticles are known for their robust structural integrity, stability under storage conditions, and controlled release capabilities.16 In spite of their excellent carrier properties are known, GMT nanoformulations are characterized by low drug loading (DL%, amount of drug per amount of lipid formulation) and low entrapment efficiency (EE%, extent of drug that is encapsulated within the nanocarrier).17 Additionally, liposomes face challenges for the reduced physical stability under storage conditions.18 Metal cations have frequently been utilized in nanoformulations due to their ability to enhance drug loading through drug complexation.19 For instance, Vyxeos was approved in 2017, a multilamellar lipid formulation that uses copper complexation for enhancing loading of cytarabine (which has a similar chemical structure to GMT) and daunorubicin.19 Starting from these premises, the goal of this research is to study the interaction between GMT and biorelevant metal cations in order to propose metal complexation as a potential strategy for the development of new GMT nanoformulations. Mn2+, Zn2+, and Ca2+, commonly present in biological fluids, are proposed as candidates for their importance in many physiological activities,20−22 and for their ability to modulate the tumor microenvironment by inducing M1 polarization (Mn2+) in tumor-associated macrophages,23 activating the Wnt-3a/β-catenin signaling pathway (Zn2+) in cancer cells,24 and treating the hepatocarcinogenesis (Ca2+).25 Moreover, Mn2+, Zn2+, and Ca2+ are clinically relevant, being used as adjuvants all via systemic administration.26−28

First, the acid–base behavior of GMT was investigated by performing spectrophotometric titrations on GMT solutions under various temperature conditions (15, 25, 37, and 45 °C). Then, formation constants of Mn2+, Zn2+, and Ca2+–GMT species were obtained by using above all potentiometric, spectrophotometric, and 1H NMR titrations. Also, the efficacy of M2+–GMT (metal–GMT) vs free GMT and free M2+ in promoting cell death was tested in vitro in a murine osteosarcoma cell line (K7M2). Furthermore, a series of computational investigations were carried out by using traditional density functional theory calculations under implicit solvation as well as via state-of-the-art spin-polarized ab initio molecular dynamics simulations with explicitly treated solvent molecules at a finite temperature to investigate the molecular modalities of interaction of Mn2+–GMT species as well as the structure of the liquid environment around GMT and Mn2+–GMT species. The reported results led to the identification of optimal conditions for pH, temperature, and the drug-to-metal molar ratio that maximize M2+–GMT interactions. These findings will pave the way for GMT nanoformulations with desirable quality attributes for the treatment of various cancers.

Materials and Methods

Materials

Manganese, zinc, and calcium solutions were obtained by weighing and dissolving the corresponding salt manganese(II) sulfate monohydrate (Sigma-Aldrich, purity >99%), zinc(II) chloride eptahydrate (Sigma-Aldrich, puriss.) and calcium(II) chloride dihydrate (Fluka, purity >99%). All metal cation solutions were standardized by titration with EDTA (ethylenediamine tetraacetic acid disodium salt, Sigma-Aldrich, purity ≥99%) standard solution. GMT solutions were prepared by weighing and dissolving GMT hydrochloride (TCI, purity >98%). Solutions of hydrochloric acid and sodium hydroxide were obtained by dilution of Fluka ampules, subsequently standardized with dried sodium carbonate (Sigma-Aldrich, purity ≥99.5%), and potassium biphthalate (Sigma-Aldrich, purity ≥99.5%), respectively. Solutions of sodium hydroxide were frequently prepared and stored in bottles with soda lime traps. Solutions of sodium chloride were obtained by weighing the corresponding salt (Sigma-Aldrich, puriss.), previously dried in an oven at 110 °C. Distilled water (conductivity <0.1 μS cm–1) and grade A glassware were employed for the preparation of all the solutions. Phosphate buffer saline (PBS, 1X), Dulbecco’s Modified Eagle’s Medium (DMEM), and trypsin-EDTA solution (0.25% trypsin and 0.53 mM EDTA) were purchased from Life Technologies. Fetal bovine serum (FBS) was purchased from Atlanta Biologicals (Flowery Branch, GA). Amicon Ultra-4 centrifugal filter device (MWCO = 10 kDa) was purchased from EMD Millipore (Billerica, MA). Tissue culture flasks (T25 and T75) were purchased from VWR International (Radnor, PA). Round bottom 96-well plates (Cellstar) were purchased from Greiner Bio-One (Kremsmünster, Austria).

Potentiometric Apparatus and Procedure

Two distinct systems were employed for the potentiometric titrations, both arranged in an identical configuration of an automatic dispenser Metrohm Dosino 800, a Metrohm model 809 Titrando potentiometer, and a Metrohm LL-Unitrode WOC combined glass electrode. Each potentiometric system was connected to a PC, and the experimental titration data were acquired by Metrohm TIAMO 2.2 software. The estimated accuracy of both systems is ±0.15 mV for the emf and ±0.002 mL for the titrant volume. Each titration consists in additions of volumes of NaOH standard to 25 mL of the solution containing GMT, HCl and a supporting electrolyte (NaCl) for the acid–base study and M2+ salt (M2+ = Mn2+ or Zn2+ or Ca2+), GMT, HCl and NaCl for the complexation study. Experimental details on the potentiometric titrations are reported in Table 1. For all measurements, glass jacket thermostated cells were used to perform titrations under different temperatures (15 ≤ t/°C ≤ 45). In addition, pure N2 was bubbled to remove CO2 and O2. For each measurement, an independent titration of HCl with standard NaOH was performed to calculate the standard electrode potential E0 and the pKw value under the same experimental ionic strength and temperature.

Table 1

Experimental Conditions for Potentiometric, Spectrophotometric, and NMR Titrations (pH Range 2–10)
techniquet °CCMa mmol L–1CGMTb mmol L–1M/GMT ratio
potentiometry15, 25, 37, 450.50.51:1
0.50.751:1.5
0.511:2
10.52:1
spectrophotometry25, 37, 45 0.04–0.08 
450.020.041:2
450.0350.051:1.4
450.020.081:4
450.040.041:111
1H NMR25 5 
 25651.2:1
aMetal cation concentration.
bGMT concentration.

UV–Vis Apparatus and Procedure

The spectrophotometric titrations were carried out by a Varian Cary 50 UV–vis spectrophotometer equipped with an optical fiber with a fixed 1 cm path length, interfaced to a PC by the Varian Cary WinUV software, and an automatic dispenser Metrohm 715 Dosimat. For each titration point, the couple data of absorbance (Abs) and pH vs volume of titrant (mL) were recorded simultaneously by using a Metrohm glass electrode and a Metrohm-713 pH meter. All titrations were performed using a thermostated cell to keep the desired temperature under N2 to remove CO2 and O2 from the solutions. The experimental conditions of the titrations are listed in Table 1. For each system, at least four measurements were performed, scanning the spectral range 225 nm ≤ λ ≤ 350 nm in different conditions of GMT concentration and metal–GMT (M/GMT) ratio. Before each measurement, a baseline containing only HCl, NaCl, and H2O was recorded to subtract the matrix contribution.11

1H NMR Apparatus and Procedure

The spectrometer employed for the collection of 1H NMR spectra was a Varian 500 FT-NMR. 1,4-dioxane was used as internal reference (δCHdioxane = 3.70 ppm), and the chemical shifts are referred to the tetramethylsilane (TMS). All the measurements were carried out in a 9:1 H2O/D2O solution of GMT or Zn2+–GMT at t = 25 °C, suppressing the water signal by the presaturation technique. Experimental details on NMR titrations are reported in Table 1.

Cell Culture

104 cells/mL of K7M2-WT were plated in 25 cm2 cell culture flasks and cultured by DMEM with 10% FBS and 1% PS. The cells were grown in a Thermo Scientific CO2 incubator at 37 °C and 5% CO2. The medium was exchanged every 2/3 days. The cells were split as they reached ca. 80–90% of confluency.

K7M2-WT Cell Viability

K7M2-WT cells were seeded in 96-well plates at a density of 104 cells per well. The cells were allowed to grow (DMEM + 10% FBS + 1% PS) for 24 h at 37 °C and 5% CO2. The drug solution was added in each well at different GMT or GMT-equivalent concentrations between 0.01 and 2500 nmol L–1. After the treatment, the cells were incubated for an additional 48 h under 5% CO2 at 37 °C in DMEM + 10% FBS + 1% PS. Cell viability was analyzed using MTT (3-(4, 5-dimethylthiazolyl-2)-2, 5-diphenyltetrazolium bromide) assay (Invitrogen, Thermo-Fisher Scientific). Briefly, the cells were rinsed twice with PBS and 110 μL of 1.09 mmol L–1 MTT (in PBS) was added to each well, followed by plate incubation for 4 h at 37 °C and 5% CO2. Subsequently, 80 μL of solution was removed from each well and 55 μL of DMSO (dimethylsulfoxide) was added and mixed. The plates were, then, covered and incubated at 37 °C for an additional 10 min. The absorbance was read at 540 nm using a multimode microplate reader (Synergy H1, Biotek). Cell viability was calculated as follows.

equation image

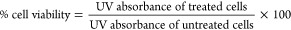
Postprocessing Calculations

Protonation constant values, speciation model for each metal-complex system, formation constant values, standard potential E0, analytical concentration of the reagents, and junction potential of each titration were determined by processing potentiometric results by the BSTAC4 and STACO4 programs. The parameters for the dependence of complex formation constants on temperature were obtained using LIANA. More details on the software employed in the refinement of the experimental data are reported in ref (29). For 1H NMR titrations, the HypNMR software was employed to obtain protonation and formation constant values, as well as the individual chemical shift of each species, using the observed signals and assuming fast mutual exchange in the NMR time scale.30 HypSpec program was used for UV data, enabling us to refine protonation and formation constants as well as the molar absorption coefficient values (ε) of all species. The HySS program was used to obtain the speciation diagrams and the formation percentages of the complex species.31

Density Functional Theory Calculations and Ab Initio Molecular Dynamics

All static calculations were performed by means of the Gaussian 16 software.32 DFT was used to evaluate the ground-state structures of the selected molecular species. In this work, the B3LYP33 hybrid exchange and correlation functional, with 100% of exact exchange, was employed. Geometry optimizations of the molecular structures were performed under implicit solvation conditions using the 6-311++G(d,p) atomic basis set for all of the atoms. In addition, when the manganese cation was included, the Stuttgart–Dresden (SDD) basis set was employed for this species. The latter basis set treats the inner core electrons with a constant pseudopotential, and the valence electrons with a triple-ζ valence basis set. We employed this strategy to enhance the computational efficiency when describing transition metals. The conductive polarizable continuum model (CPCM)34 with typical parameters was employed for water.

Whereas GMT is neutral and typically found in a singlet electronic state, when GMT binds the [MnOH]+ cation, the formed complex is an open-shell species with a global positive charge of +1 lying in a doublet spin state. Unrestricted spin-polarized DFT calculations were performed in those cases. Born–Oppenheimer AIMD simulations were performed by using the CP2K35 software suite on two different numerical samples. The first one contained one GMT and 142 H2O molecules (i.e., 455 atoms), whereas the second one was composed of one GMT+[MnOH]+ complex surrounded by 143 water molecules (i.e., 461 atoms). While in the former case, a neutral simulation box with a global spin component equal to one was simulated, a simulation box having a global charge equal to +1 and a spin component of 2 was imposed in a spin-polarized AIMD simulation. Initial structures of GMT and GMT+[MnOH]+ were preoptimized at the B3LYP/6-311++G(d,p) level with the CPCM water as an implicit solvation model. As for the GMT+[MnOH]+, in light of the observed higher stability with respect to other plausible molecular arrangements, only the complex with the [MnOH]+ moiety chelated between the two hydroxyl groups of GMT was simulated via spin-polarized AIMD. Cubic supercells with edges of 16.22 and 16.85 Å were built for the systems containing the neutral GMT structure and the GMT+[MnOH]+ complex, respectively. Periodic boundary conditions were applied along the three Cartesian axes to mitigate spurious finite-size effects. During AIMD simulations, wave functions of each atomic species were expanded in double-zeta-valence-plus-polarization (DZVP) basis sets with the Goedecker–Teter–Hutter pseudopotentials36 using the GPW method.37,38 A plane-wave cutoff of 400 Ry was adopted. Exchange and correlation effects were treated via the PBE39 density functional, belonging to the Generalized Gradient Approximation (GGA) DFT class. Moreover, to take into account dispersion interactions, the dispersion-corrected PBE+D340,41 functional was employed. Each trajectory was simulated for 15 ps at a nominal temperature of 330 K. A temperature higher than the room one was selected to mitigate the well-known overstructuring of the solvation environment introduced by GGA DFT functionals in liquid water and for more closely mimicking the operational conditions of the experiments. The temperature was kept fixed via a CSVR42 thermostat set with a time constant equal to 50 fs. In this way, the systems were simulated in an isothermal-isochoric (NVT) ensemble, whereas the nuclei dynamics were classically propagated through the Verlet algorithm with a time-step of 0.5 fs.

Results and Discussion

Acid–Base Behavior

A preliminary acid–base study of GMT was performed to assess its protonation constant values, which were needed for the subsequent metal–ligand investigation. The values of the protonation constant of the amine group in the 4′ position in GMT, determined at different temperatures (15, 25, 37, and 45 °C), are listed in Table 2. To the best of our knowledge, the only related data present in the literature refers to GMT’s log K = 3.60 at t = 25 °C and unspecified ionic strength.43 This value coincides with that at t = 25 °C and I = 0.1 mol L–1 determined by us and reported in the current study.

Table 2

Experimental Protonation and Formation Constants of H+–GMT, Zn2+–GMT, Mn2+–GMT, and Ca2+–GMT Species at I = 0.1 mol L–1
reactiont /°Clog β ± SDa
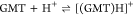An external file that holds a picture, illustration, etc. Object name is am4c12550_m003.gif153.94 ± 0.03
253.60 ± 0.02
373.51 ± 0.02
453.55 ± 0.02
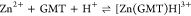An external file that holds a picture, illustration, etc. Object name is am4c12550_m004.gif257.64 ± 0.04
377.55 ± 0.04
457.63 ± 0.06
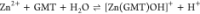An external file that holds a picture, illustration, etc. Object name is am4c12550_m005.gif25–5.00 ± 0.04
37–4.73 ± 0.04
45–4.40 ± 0.03
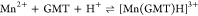An external file that holds a picture, illustration, etc. Object name is am4c12550_m006.gif256.39 ± 0.03
376.50 ± 0.01
457.24 ± 0.08
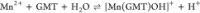An external file that holds a picture, illustration, etc. Object name is am4c12550_m007.gif25–6.52 ± 0.02
37–6.15 ± 0.07
45–5.55 ± 0.08
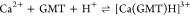An external file that holds a picture, illustration, etc. Object name is am4c12550_m008.gif256.79 ± 0.05
376.64 ± 0.09
456.38 ± 0.03
reactiont /°Clog K
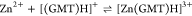An external file that holds a picture, illustration, etc. Object name is am4c12550_m009.gif254.04
374.04
454.08
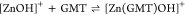An external file that holds a picture, illustration, etc. Object name is am4c12550_m010.gif254.14
374.05
454.38
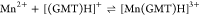An external file that holds a picture, illustration, etc. Object name is am4c12550_m011.gif252.79
372.99
453.69
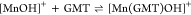An external file that holds a picture, illustration, etc. Object name is am4c12550_m012.gif253.94
373.05
454.16
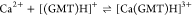An external file that holds a picture, illustration, etc. Object name is am4c12550_m013.gif253.19
373.13
452.83
aSD = standard deviation.

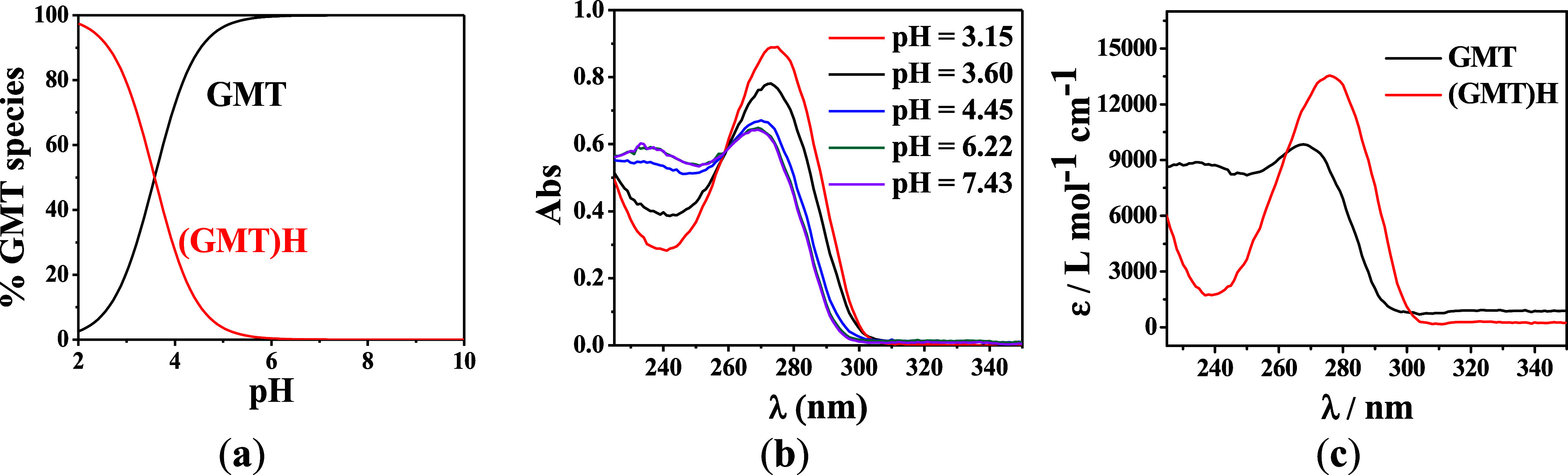
The speciation diagram of GMT obtained at t = 37 °C and I = 0.1 mol L–1 is reported in Figure ​Figure22a. The diagram shows that at pH = 2.0, almost 100% of GMT is protonated. At pH = 6.0, all of the ligand is deprotonated. UV spectra obtained at selected pH are reported in Figure ​Figure22b. A maximum of absorption is distinguishable at λ = 270 nm, which shows a blueshift in the spectrum as a consequence of pH increase. From pH = 3.15 to 6.22, a hypochromic effect is observed, but no changes are found in the pH range 6.22 ≤ pH ≤ 7.43. An isosbestic point at λ = 260 nm, representing GMT/[(GMT)H]+ equilibrium, is also evident. The molar extinction coefficients (ε) vs. wavelength (λ) of GMT species are reported in Figure ​Figure22c. More in detail, two maxima of 14 000 L mol–1 cm–1 at λ = 280 nm and 9 500 L mol–1 cm–1 at λ = 275 nm are observed for [(GMT)H]+ and GMT species, respectively.

An external file that holds a picture, illustration, etc. Object name is am4c12550_0002.jpg

(a) Speciation diagram of GMT species; (b) UV spectra of GMT solution at selected pH values at CGMT = 0.06 mmol L–1; (c) molar extinction coefficients (ε) vs wavelength (λ). Experimental conditions: t = 37 °C, I = 0.1 mol L–1 in NaCl.

Mn2+–GMT, Zn2+–GMT, and Ca2+–GMT Complexes

The study of the interaction between GMT and Mn2+, Zn2+, and Ca2+ was performed with the aim to use metal complexation for altering the physiochemical properties of GMT leading to the development of enhanced GMT nanoformulations in lipid or polymeric matrices. Once the acid–base properties of the ligand as well as the hydrolytic behavior of the metal cations (hydrolytic constants reported in Tables S1–S3 of Supporting Information) were determined, we then investigated the interaction between GMT and metal cations. With the aim of choosing the most reliable speciation model, potentiometric titrations were performed on solutions with different metal–ligand ratios.

All the experimental formation constant values of the GMT complex species under different temperature conditions are reported in Table 2. To have an immediate comparison between the stability of the different species, the partial formation constants (log K) were also calculated and are reported in Table 2. All the protonation and formation constant values, listed in Table 2, were calculated by fitting the experimental potentiometric data with BSTAC4 and STACO4 programs.

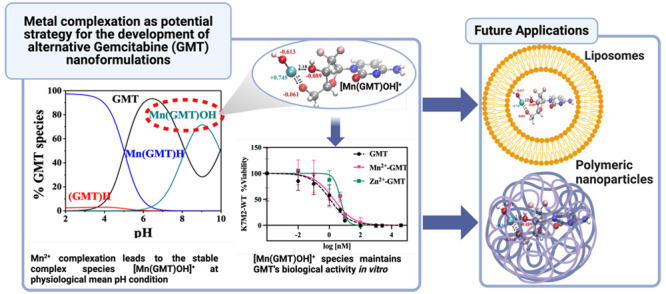
For all the systems, the most reliable speciation model was selected by doing several trials on potentiometric titrations with different metal–ligand ratios, testing different models, and choosing the one that met criteria such as simplicity, lowest mean deviation parameters, and higher formation percentages of the species, as indicated in the ref (44). More in detail, for Mn2+–GMT and Zn2+–GMT the same speciation pattern was found, which includes [M(GMT)H]3+ (resulting from the protonation of GMT and subsequent interaction with the free metal cations) and [M(GMT)OH]+ species (formed by the interaction of the hydrolytic species of the metal cations and free GMT). A simpler speciation model has emerged for Ca2+–GMT, with the latter showing the formation of the [Ca(GMT)H]3+ species only. As shown in Figure ​Figure33a, at t = 45 °C, the [Mn(GMT)H]3+ species, found in the range 2 ≤ pH ≤ 6, reaches its maximum formation percentage, corresponding to 98% at pH = 2. [Mn(GMT)OH]+ species, formed in the range 7 ≤ pH ≤ 10, reaches 70% at pH = 9. This complex formation reduces the [MnOH]+ percentage in solution, which reaches a maximum of 10% at pH 10. Figure ​Figure33b shows the speciation of the Zn2+–GMT system at t = 45 °C, where the [Zn(GMT)H]3+ complex exists in the range 2 ≤ pH ≤ 6, reaching a maximum of 98% at pH = 2. On the other hand, the [Zn(GMT)OH]+ species forms at 5.5 ≤ pH ≤ 10, with a maximum fraction equal to 95% at pH = 8. The hydrolytic species [ZnOH]+, due to the formation of a high amount of the complex species, reaches a maximum of 2% at pH = 8.5. As displayed in Figure ​Figure33c, [Ca(GMT)H]3+ species is formed in the range 2 ≤ pH ≤ 5 with a maximum of 31% at pH = 2 at t = 45 °C. The weak hydrolytic species [CaOH]+ does not form a significant percentage in the investigated pH range, so it is not present in the reported speciation diagram. By comparing the log K value reported in Table 2, both Mn2+–GMT and Zn2+–GMT systems exhibit a higher stability at t = 45 °C than t = 37 °C or t = 25 °C. Conversely, the Ca2+–GMT system shows better stability at physiological temperature conditions (37 °C). Moreover, according to these preliminary results, Mn2+ and Zn2+ interactions lead to more stable complex species than Ca2+, as already observed in several other studied systems.45,46 Also, t = 45 °C, 1:1 = M/GMT molar ratio, and pH = 7.4 (mean physiological pH) for Mn2+ and Zn2+, may be suitable to prepare nanoformulations as we can achieve higher formation percentages of metal complexes under those conditions.

An external file that holds a picture, illustration, etc. Object name is am4c12550_0003.jpg

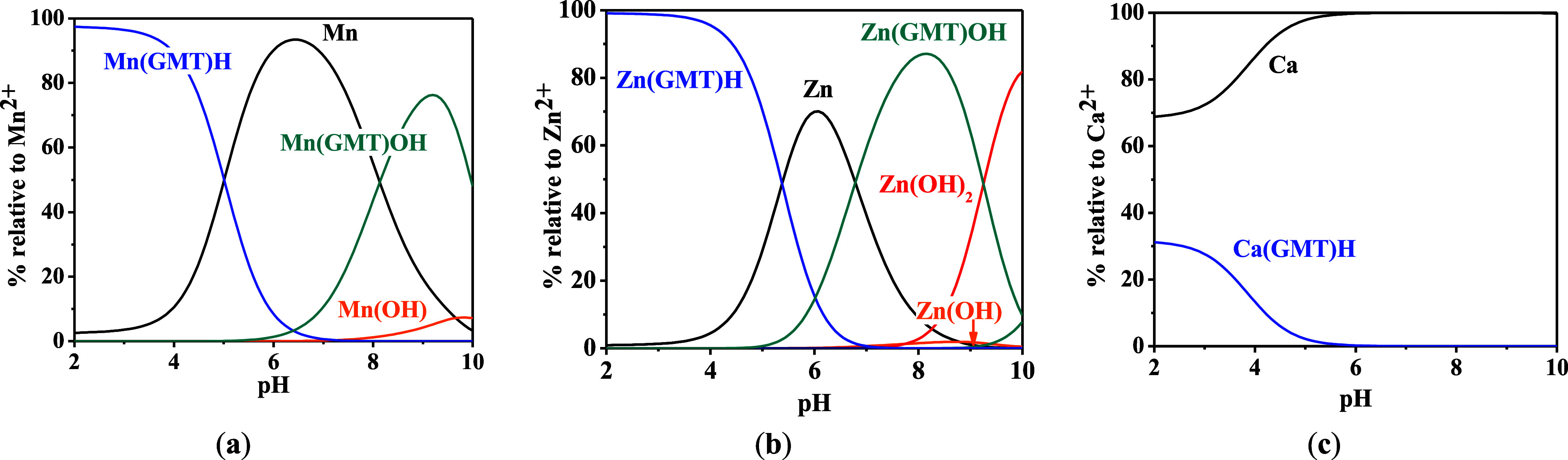
Distribution diagrams of (a) Mn2+–GMT, (b) Zn2+–GMT and (c) Ca2+–GMT systems at t = 45°, CGMT = CM = 1 mmol L–1 and I = 0.1 mol L–1.

The two systems Mn2+–GMT and Zn2+–GMT were also investigated by spectrophotometric titrations to confirm the formation constant values and the speciation models and to examine the spectral behavior of the complex species. A comparison between the formation constants determined by potentiometry and spectrophotometry at t = 45 °C is reported in Table 3. The formation constant values obtained by spectrophotometry agree with those obtained by potentiometry.

Table 3

Comparison between Experimental Formation Constants Obtained by Potentiometry, UV Spectrophotometry, and 1H NMR Spectroscopy at t = 45°C and I = 0.1 mol L–1
reactionlog βUV ± SDalog βpotentiometry ± SDalog βNMR ± SDa
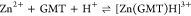An external file that holds a picture, illustration, etc. Object name is am4c12550_m014.gif7.63 ± 0.077.63 ± 0.067.63 ± 0.08
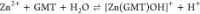An external file that holds a picture, illustration, etc. Object name is am4c12550_m015.gif–4.49 ± 0.04–4.40 ± 0.03–4.24 ± 0.08
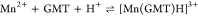An external file that holds a picture, illustration, etc. Object name is am4c12550_m016.gif7.36 ± 0.087.24 ± 0.08 
An external file that holds a picture, illustration, etc. Object name is am4c12550_m017.gif–5.62 ± 0.09–5.55 ± 0.08 
aSD = standard deviation.

Figure ​Figure44 displays the UV spectra for free GMT and M2+–GMT systems at pH values of 3.5 and 7.4. For both systems, a hyperchromic effect is observed at these pH values, indicating the formation of a complex species. Specifically, (a) the absorbance change (ΔAbs) is 0.14 at λ = 270 nm and pH 3.5, and ΔAbs = 0.09 at λ = 280 nm and pH 7.4; (b) ΔAbs is 0.12 at λ = 270 nm and pH 3.5, and ΔAbs = 0.07 at λ = 280 nm and pH 7.4.

An external file that holds a picture, illustration, etc. Object name is am4c12550_0004.jpg

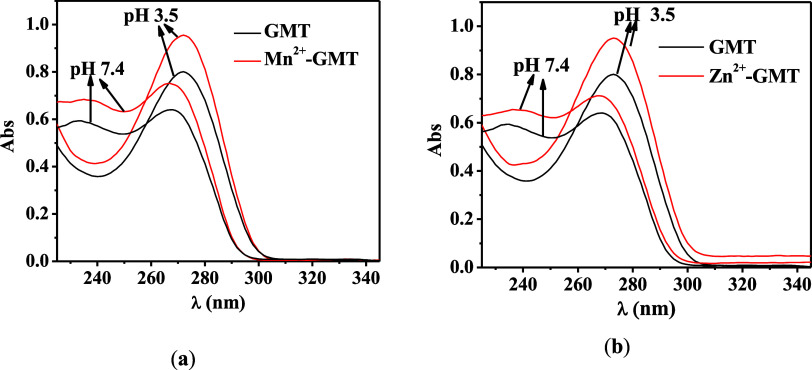
UV spectra at selected pH of (a) Mn2+–GMT and (b) Zn2+–GMT at t = 45 °C, I = 0.1 mol L–1, and CGMT = CM = 0.04 mmol L–1.

In Figure S2 (Supporting Information), some of the spectra acquired on solutions containing Mn2+–GMT and Zn2+–GMT at the selected pH are reported.

As far as we know, there are no stability data in the literature regarding the formation of gemcitabine complexes with Ca2+, Mn2+, or Zn2+ metal cations. However, it is possible to consider cytidine, as the gemcitabine molecule is a deoxycytidine where the fluorine atoms replaced the hydrogen atoms on the 3 carbon (see Figure ​Figure55). In the case of cytidine, there are some papers in the literature, albeit dated, on the complexation with Mn2+ and Zn2+47,48 Reddy and Rao report the formation constants of the species at t = 35 °C and I = 0.1 mol L–1 in KNO3. For Mn2+-cytidine and for Zn2+-cytidine the speciation models include only the ML species with stability constants equal to 2.51 and 2.60, for Mn2+ and Zn2+, respectively.47 Although the speciation models and ionic media differ and GMT and cytidine are not identical, the stability of the species remains comparable, particularly for Mn2+. For example, logK for [Mn(GMT)H]3+ species is 2.99 at I = 0.1 mol L–1 in NaCl and t = 37 °C (see Table 2). In the literature, referring to cytidine-5′-triphosphoric acid, a paper reports the IUPAC evaluation on stability data of species formed by this ligand with Mn2+ and Zn2+ at t = 25 °C and I = 0.1 mol L–1 in R4NX.48 The speciation model for both Mn2+ and Zn2+ includes two species, namely, ML and MLH. The logK values referring to the species common to the literature model and ours, that is MLH, are 3.0 and 2.88 for Mn2+ and Zn2+, respectively. The value here reported for the gemcitabine species with Mn2+, having the same stoichiometry and the same formation reaction, under the same temperature and ionic strength in a different ionic medium (NaCl), is logK = 2.79. This value is fairly close to that of cytidine-5′-triphosphoric acid.

An external file that holds a picture, illustration, etc. Object name is am4c12550_0005.jpg

1H NMR spectra on solutions containing GMT at C = 5 mmol L–1, t = 25 °C, I = 0.1 mol L–1 in NaCl, and 2.72 ≤ pH ≤ 8.52.

Several 1H NMR titrations were performed on GMT solutions. All 1H NMR spectra at selected pH values and t = 25 °C show seven signals, as displayed in Figure ​Figure55. Two double doublets at 3.8 and 3.9 ppm related to CH2 protons, two multiplets at 4.1 and 4.3 ppm assigned to the CH-4 and CH-5, respectively, a multiplet at 6.1 ppm of the CH-2, and two doublets at 6.2 and 7.9 ppm related to the CH-5′ and CH-6′ protons. By increasing the pH value, an upfield of 0.2 ppm of the CH-5′ and CH-6’ protons chemical shift can be observed due to the deprotonation of the amine group of the pyrimidine ring.

The Zn2+–GMT system was also investigated via 1H NMR titrations. The same trend of free GMT was found for the Zn2+–GMT system (Figure S3 in Supporting Information). The analysis of the NMR experimental data confirms the speciation model of the Zn2+–GMT system and allows to obtain the formation constant values of both species, despite the chemical shift differences referred to GMT and GMT with Zn2+ are very small (for CH-5′ and CH-6′ protons Figure S4 in Supporting Information). This is often observed for weak interactions in aqueous solutions and when the species, involved in the equilibria, are rapidly exchanging on the NMR time scale. The formation constant values, reported in Table 3, obtained via 1H NMR spectroscopy closely match those gathered via potentiometry and UV spectrophotometry (no statistical differences have been found, p = 0.8333, One-way ANOVA). By the determination of the protonation and formation constants under different temperatures, listed in Table 2, it is possible to calculate the protonation and formation enthalpy change values. More in detail, the protonation and formation constants obtained by the potentiometric titrations under various temperatures (calculated by BSTAC4 and STACO4 programs), were processed using the LIANA fitting program, and taking into account the following van’t Hoff equation49

equation image

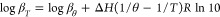
where log βT is the stability constant at a certain temperature (expressed in Kelvin), log βθ is the stability constant at T = 298.15 K, and R is the universal gas constant expressed as 8.314 J K–1 mol–1. The values of protonation and formation enthalpy changes of GMT and M2+–GMT species are collected in Table 4, together with the entropy and free energy values. All formation reactions exhibit negative changes in free energy, indicating that they can occur spontaneously

Table 4

ΔG, ΔH, and TΔS of GMT, Zn2+–GMT, Mn2+–GMT, and Ca2+–GMT Species at t = 25°C and I = 0.1 mol L–1 in NaCl
reactionΔGaΔH ± SDa,bTΔSa
An external file that holds a picture, illustration, etc. Object name is am4c12550_m018.gif–20.5–20 ± 81
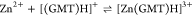An external file that holds a picture, illustration, etc. Object name is am4c12550_m019.gif–23.122 ± 545
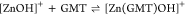An external file that holds a picture, illustration, etc. Object name is am4c12550_m020.gif–23.644 ± 568
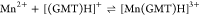An external file that holds a picture, illustration, etc. Object name is am4c12550_m021.gif–15.988 ± 7104
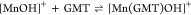An external file that holds a picture, illustration, etc. Object name is am4c12550_m022.gif–22.510 ± 632
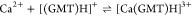An external file that holds a picture, illustration, etc. Object name is am4c12550_m023.gif–18.2–11 ± 37
aIn kJ mol–1.
bSD = standard deviation.

In Vitro Evaluation Activity

GMT is known for its efficacy in treating various cancers either alone or in combination with other drugs,3 thus representing a promising therapeutic agent. To assess the biological activity of M2+–GMT complex species, specifically [Mn(GMT)OH]+ and [Zn(GMT)OH]+, the cell kill ability was investigated in vitro on K7M2-WT cells, an established model of murine osteosarcoma. Ca2+–GMT system’s toxicity was not investigated as, based on our speciation study, it did not show any complex species under physiological pH conditions (pH = 7.4). These investigations aimed to determine whether the cytotoxic effect observed with free GMT is preserved after complexation. The results, depicted in Figure ​Figure66, illustrate that both Mn2+ and Zn2+ exhibit toxicity against K7M2-WT cells, with different potency (Mn2+ > Zn2+). Moreover, Mn2+–GMT displays an IC50 value of 1 nM, as for free GMT (1 nM), suggesting that the complexation with Mn2+ did not alter GMT’s biological activity. Conversely, Zn2+–GMT exhibits reduced cytotoxicity with an IC50 value of 5 nM, indicating a potency decrease of at least 5-fold compared to that of the free drug. At physiological pH, the concentration of [Zn(GMT)OH]+ species is higher compared to [Mn(GMT)OH]+, resulting in a lower amount of free GMT and a reduced amount of free metal cation (25% in the Zn2+–GMT versus 45% in Mn2+–GMT, at t = 37 °C). Consequently, this reduction in activity is likely attributable to the lower biological activity of Zn2+ compared to Mn2+ and/or the reduced availability of free GMT and free metal cation. These findings highlight the critical role of the metal ion selection in maintaining or altering GMT’s therapeutic efficacy. We are currently evaluating the in vivo antitumor efficacy of M2+–GMT complexes for further elucidating their potential as novel cancer therapies. These efforts will aim to translate the promising cytotoxicity results observed in vitro into effective treatments for cancer patients.

An external file that holds a picture, illustration, etc. Object name is am4c12550_0006.jpg

Viability of K7M2-WT at 48 h after exposure to therapy. IC50: GMT 1 nM (95% CI of 1 to 2 nM); Mn2+ 5 nM (95% CI of 3 to 7 nM), Zn2+ 11 nM (95% CI of 8 to 20 nM), Mn2+–GMT 1 nM (95% CI of 1 to 4 nM), and Zn2+–GMT: 5 nM (95% CI of 4 to 6 nM). Data were calculated using a nonlinear regression log(inhibitor) vs response (variable slope). n = 4/concentration.

Density Functional Theory Calculations and Ab Initio Molecular Dynamics

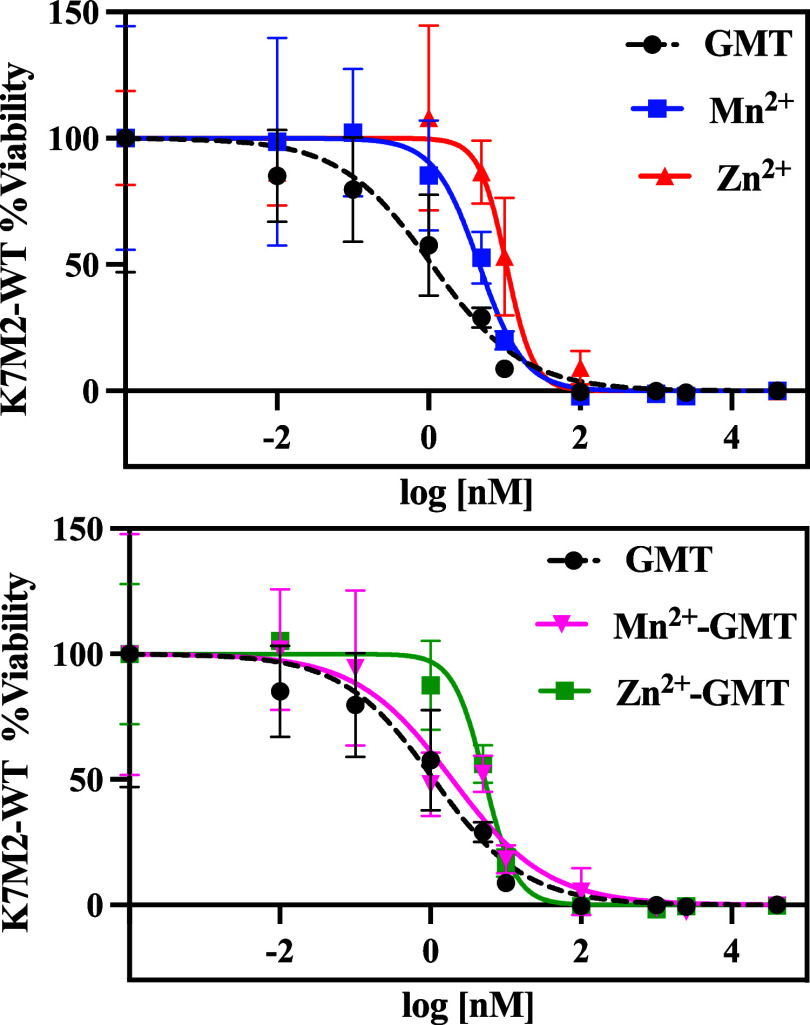
The [Mn(GMT)OH]+ complex species, which exhibited higher efficacy in vitro, was thoroughly investigated not only by means of traditional DFT calculations but also by invoking state-of-the-art spin-polarized AIMD simulations where the active role of the solvation is explicitly and dynamically considered. To benchmark the quality of the adopted DFT framework, we report in Figure ​Figure77a the molecular structure of neat GMT optimized at the B3LYP/6-311++G(d,p) level of theory in the presence of the model solvent CPCM mimicking the surrounding water environment. The structure significantly deviates from its gas-phase ground-state configuration. Specifically, under gas-phase conditions using the B3LYP/6-311++G(d,p) level of theory, an internal hydrogen bond forms between the hydroxyl group of the ribose ring and the nearest oxygen atom.50 However, upon inclusion of implicit water solvent, such an internal bond disappears (Figure ​Figure77a). Despite the absence of this stabilizing intramolecular interaction, the structure’s ground-state energy under implicit solvation (Figure ​Figure77a) is lower than its gas-phase counterpart by approximately 12.2 kcal/mol (i.e., −1014.740293 vs −1014.720866 a.u).50 Furthermore, the lack of the internal hydrogen bond results in a less asymmetric distribution of partial charges on the oxygen atoms of the hydroxyl groups in GMT. In the presence of the CPCM solvent model (Figure ​Figure77a), the absolute charge difference is |−0.295 + 0.262| = 0.33e–. In contrast, this difference nearly doubles under gas-phase conditions, totaling |−0.238 + 0.182| = 0.56e–.50 Another computational study,51 using the more accurate M06 exchange and correlation functional and a smaller basis set (6-311G) under implicit solvation, reports a symmetric distribution of charges around these oxygen atoms, with a minimal absolute difference of |−0.276 + 0.272| = 0.006e–. Similar small discrepancies are noted for the nitrogen atom of the amine group: our B3LYP/6-311++G(d,p) calculations with CPCM solvation yield a partial charge of −0.343e– (Figure ​Figure77a), whereas other studies report local Mulliken charges of −0.29850 and −0.390e–51 at the same level of theory (B3LYP/6-311++G(d,p))50 and under solvation with different methods (M06/6-311G with PCM).51 These findings show that the qualitative behavior of the electron density appears to be consistent over different DFT approaches and basis sets but preliminarily highlights important differences introduced by the inclusion, even though in an implicit manner, of the solvation environment in determining the molecular structure of GMT.

An external file that holds a picture, illustration, etc. Object name is am4c12550_0007.jpg

Molecular structure optimized at the B3LYP/6-311++G(d,p) level of theory under CPCM water implicit solvation of GMT (a), GMT with [MnOH]+ chelated by the two hydroxyl groups (b) and GMT with [MnOH]+ bound at the amine group (c). Colored values are the Mulliken partial charges associated with the closest atom of the same color, whereas numbers in black refer to the distance displayed by the arrows in Å.

The speciation curves reported in Figure ​Figure33a, show that the GMT+[MnOH]+ complex, i.e., [Mn(GMT)OH]+, forms starting from pH ≈ 6. To investigate its molecular structure, we executed a series of unrestricted B3LYP/6-311++G(d,p) calculations under implicit solvation conditions. The two molecular sites of GMT at which the [MnOH]+ species can a priori be chemisorbed are (i) in the region between the two hydroxyl groups (site1) and (ii) in the proximity of the amine group of the pyrimidine ring (site2), whose ground-state structures are reported in Figure ​Figure77b,c, respectively. During the chelation process of [MnOH]+ at the site1 most of the negative charge characterizing the oxygen atoms of the hydroxyl groups of GMT is transferred to the oxygen atom of [MnOH]+ and, at least in part, also to the manganese atom, which exhibits a less positive local charge than expected (+0.745e–), as shown in Figure ​Figure77b. By contrast, a significantly more positive electric charge lies on manganese (+1.063e–) when the [MnOH]+ moiety binds at the site2, as displayed in Figure ​Figure77c. This is mainly because a larger fraction of the local electron density can be donated by the two oxygen atoms of the GMT hydroxyl groups with respect to that available around the nitrogen atom of the GMT amine group. This leads to the formation of (two) shorter bonds of 2.11 and 2.18 Å between Mn and the O atoms when [MnOH]+ is chelated at site1 (Figure ​Figure77b) with respect to the minimum-energy distance of 2.24 Å featuring the bond formed between Mn and N at site2 (Figure ​Figure77c). All this evidence indicates that stronger interactions arise at the site1 than at the site2. In fact, the GMT+[MnOH]+ complex displayed in Figure ​Figure77b is 13.3 kcal/mol more stable than its counterpart reported in Figure ​Figure77c.

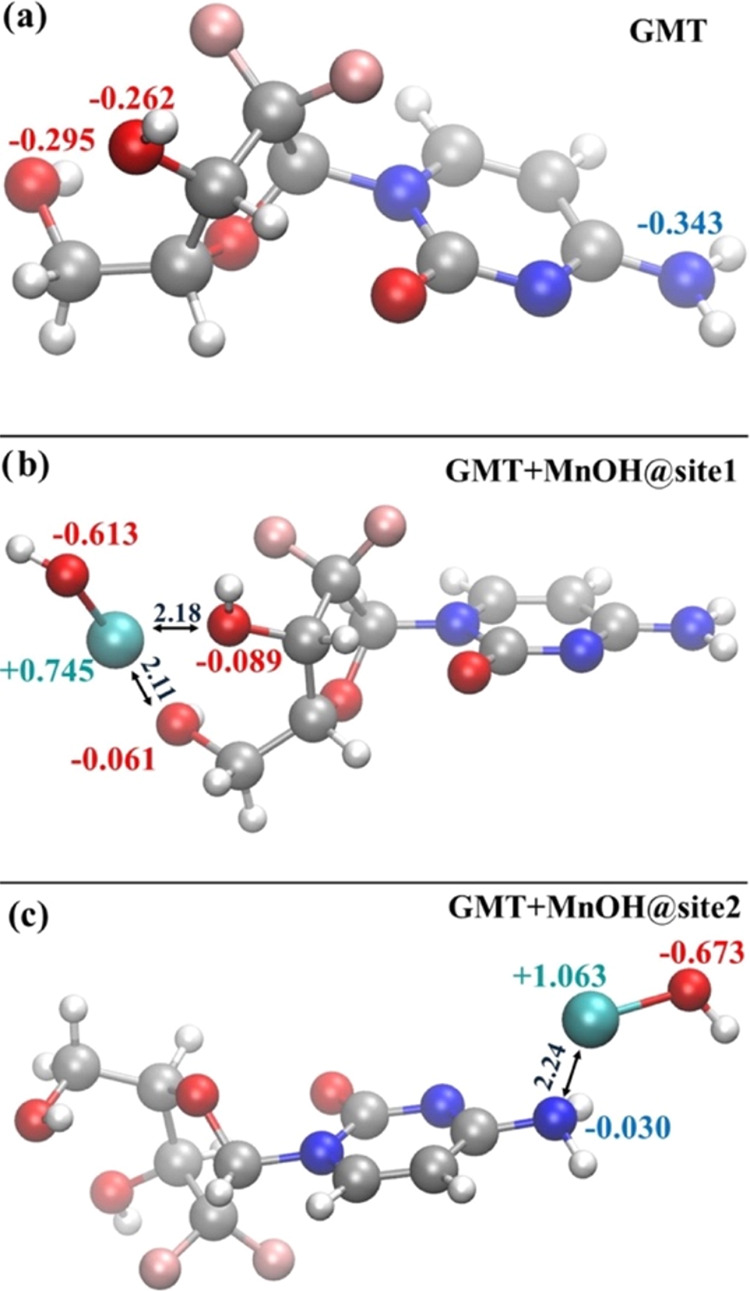
In light of the enhanced capability that [MnOH]+ exhibits in being chelated at the site1 of GMT and with the aim of exploring the role played by more realistic aqueous environments, we have performed an AIMD simulation of this GMT+[MnOH]+ structure surrounded by 143 explicitly treated H2O molecules at finite temperature. As a reference, also GMT solvated by 142 water species was simulated via AIMD. AIMD simulations of liquid systems can provide an efficient quantity that can be represented by the atomistic radial distribution functions g(r). These functions can highlight spatial correlations existing between given sets of atomic species. This way, to monitor the structure of the liquid environment around GMT, on the one hand, and of GMT+[MnOH]+, on the other hand, we determined the radial distribution function between all of the oxygen atoms of those species and the oxygen atoms of the surrounding aqueous system. As shown in Figure ​Figure88a, there exists a structuring of water molecules around the oxygen atoms of both GMT (black trace) and GMT+[MnOH]+ (red trace). Although GMT possesses 4 oxygen atoms in total, such structuring is essentially due to the two hydroxyl groups. In fact, the first peaks of those radial distribution functions are located at ≈2.8 Å, which corresponds to the typical length scale of hydrogen bonding in water under the simulated thermodynamic conditions. It is interesting to notice that when [MnOH]+ is chelated by GMT (red trace), the first peak of this radial distribution function falls at lower distances and gets narrower with respect to the case in which GMT alone is surrounded by water (black trace). This suggests that the first solvation shell around the GMT+[MnOH]+ complex is more structured and compact than the one shaping the local hydration of GMT.

An external file that holds a picture, illustration, etc. Object name is am4c12550_0008.jpg

(a) Atomistic radial distribution functions between the oxygen atoms of GMT alone and the oxygen atoms of water (black curve), the oxygen atoms of GMT while chelating the [MnOH]+ and the oxygen atoms of water (red trace), and between the manganese atom in the latter complex and the oxygen atoms of the aqueous environment (blue line). (b) Typical solvation shell around the GMT+[MnOH]+ complex at the proximity of the manganese atom extracted from ab initio molecular dynamics simulations.

By sitting on the manganese atom and by looking at the water environment, a very structurally enhanced situation emerges, as evidenced by the radial distribution function between Mn and the O atoms of water (Ow) plotted in Figure ​Figure88a (blue trace). In fact, a sharp and high first peak characterizes the correlations between those atomic species whereas the complete depletion separating the latter peak from the second one witnesses the net separation of the first and second solvation shells around the chelated Mn moiety. Moreover, the location of the first peak of the Mn–Ow radial distribution function at ≈2.0 Å demonstrates that Mn2+ establishes very strong interactions with its own hydration shell. In fact, as depicted in Figure ​Figure88b, Mn is tightly bound not only to its own OH– group and to the two chelating OH groups of GMT, but also to two additional H2O molecules stemming from the water environment and, to a lesser extent, to one of the fluorine atoms of GMT. In particular, the 3 OH– groups and one of the H2O molecules span a quite compact equatorial plane whereas out-of-plane distances of the first solvation shell of Mn2+ appear to be statistically larger. Such an octahedral-like arrangement is in line with DFT computations on Mn2+-H2O (large enough) clusters.52 All evidence emerging from our AIMD simulations magnifies the necessity of invoking an explicit description of the water environment when dealing with GMT complexes of a similar nature to those here investigated, paving the way toward a more conspicuous usage of those computational techniques in this and related research fields. Our AIMD calculations showed that the presence of solvation H2O molecules leads to the formation of a stable [Mn(GMT)OH]+ species. Thus, metal GMT complexation will represent a good strategy for enhancing the physical stability of GMT nanoformulations, thereby preventing GMT leakage from carriers and consequent reductions in drug loading (%DL) over time, while maintaining the drug’s efficacy, as confirmed by the reported MTT assays.

Conclusions

Our findings demonstrate that complex species of gemcitabine (GMT) with Mn2+ and Zn2+ (but not for Ca2+) under specific conditions achieve high formation percentages. These conditions are t = 45 °C, 1:1 molar ratio of metal-to-gemcitabine (M/GMT), and pH values of either 3 (commonly used to make liposomal formulations by the pH gradient method) or 7.4 (average physiological pH). Stabilization of [Mn(GMT)OH]+ complexes is elucidated at the molecular scale via Density Functional Theory calculations and spin-polarized ab initio molecular dynamics simulations. It turns out that the [MnOH]+ moiety is preferentially chelated by the two hydroxyl groups of GMT, whereas further stabilization of the complex is achieved by actively involving water molecules from the local hydration environment. The evidence of the significant complexing ability of GMT, mainly toward Mn2+, at t = 45 °C and pH = 3 and 7.4, and the involvement of the water molecules in conferring stability to the drug molecule will be the key factors for improving the drug loading in nanoformulations. Moreover, according to the in vitro results, GMT complexation with Mn2+ maintains the potency of the drug. The novelty of this research lies in its progression from speciation studies and computational calculations on drug-metal complexes to the concrete development of strategies for engineering new GMT liposomal and polymeric nanoparticle formulations. We are confident that our preliminary results will lead to novel GMT nanoformulations with enhanced quality attributes (better drug loading capacity and physical stability) and increased efficacy. The results reported in the current work hold the potential for achieving improved treatment outcomes, including enhanced efficacy and decreased toxicity, pivotal aspects paving the way for widespread usage of this potent therapeutic molecule.

Glossary

Abbreviations

GMTgemcitabine
FDAFood and Drug Administration
WHOWorld Health Organization
dFdCTPGMT triphosphate
dFdCDPGMT diphosphate
dFdU2′,2′-difluorodeoxyuridine
PKpharmacokinetic
EEentrapment efficacy
DLdrug loading
MTT3-(4, 5-dimethylthiazolyl-2)-2, 5-diphenyltetrazolium bromide
CPCMconductive polarizable continuum model
DFTDensity Functional Theory
AIMDAb Initio Molecular Dynamics

Supporting Information Available

The Supporting Information is available free of charge at https://pubs.acs.org/doi/10.1021/acsami.4c12550.

  • Table S1: Hydrolysis Constants of Ca2+ at different temperatures and ionic strength values; Table S2: Hydrolysis Constants of Zn2+ at different temperatures and ionic strength values; Table S3: Hydrolysis Constants of Mn2+ at different temperatures and ionic strength values; Table S4: Cartesian components of the atomic species composing the gemcitabine molecular structures shown in Figure ​Figure77 of the main text optimized at the at the B3LYP/6-311++G(d,p) level of theory under CPCM water implicit solvation. Figure S1: ε vs wavelength of (a) Zn2+-GMT and (b) Mn2+–GMT at t = 45 °C, I = 0.1 mol L–1; Figure S2: UV spectra at selected pH of (a) Mn2+–GMT and (b) Zn2+–GMT at t = 45 °C, I = 0.1 mol L–1, CGMT = 0.04 mmol L–1, CM = 0.04 mmol L–1; Figure S3: 1H NMR spectra on solutions containing Zn2+–GMT at CGMT = 5 mmol L–1 and CZn = 6 mmol L–1, t = 25 °C, I = 0.1 mol L–1 in NaCl, 2.70 ≤ pH ≤ 7.64; Figure S4. Comparison between chemical shift (δ) of (a) CH-5′ and (b) CH-6’ measured on 1H NMR spectra on solutions containing GMT and Zn2+–GMT at CGMT = 5 mmol L–1 and CZn = 6 mmol L–1, t = 25 °C, I = 0.1 mol L–1 in NaCl, 2.72 ≤ pH ≤ 8.52 (PDF)

Notes

G.C. acknowledges financial support from ICSC—Centro Nazionale di Ricerca in High-Performance Computing, Big Data and Quantum Computing, funded by European Union—NextGenerationEU— PNRR, Missione 4 Componente 2 Investimento 1.4. G.C. is thankful to CINECA for awards under the ISCRA initiative for the availability of high-performance computing resources and support.

Notes

The authors declare no competing financial interest.

Supplementary Material

References

  • Celia C.; Cristiano M. C.; Froiio F.; Di Francesco M.; d’Avanzo N.; d’Avanzo N.; Di Marzio L. Nanoliposomes as Multidrug Carrier of Gemcitabine/Paclitaxel for the Effective Treatment of Metastatic Breast Cancer Disease: A Comparison with Gemzar and Taxol. Adv. Therapeut. 2021, 4, 200012110.1002/adtp.202000121. [CrossRef] [Google Scholar]
  • Galsky M. D.; Daneshmand S.; Izadmehr S.; Gonzalez-Kozlova E.; Chan K. G.; Lewis S.; et al. Gemcitabine and cisplatin plus nivolumab as organ-sparing treatment for muscle-invasive bladder cancer: a phase 2 trial. Nat. Med. 2023, 29, 2825–2834. 10.1038/s41591-023-02568-1. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]
  • Zhao Y.; Zheng Y.; Zhu Y.; Ding K.; Zhou M.; Liu T. Co-delivery of gemcitabine and Triapine by calcium carbonate nanoparticles against chemoresistant pancreatic cancer. Int. J. Pharm. 2023, 636, 12284410.1016/j.ijpharm.2023.122844. [Abstract] [CrossRef] [Google Scholar]
  • Beutel A. K.; Halbrook C. J. Barriers and opportunities for gemcitabine in pancreatic cancer therapy. Am. J. Physiol.-Cell Physiol. 2023, 324, C540–C552. 10.1152/ajpcell.00331.2022. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]
  • Thompson B. R.; Shi J.; Zhu H.; Smith D. E. Pharmacokinetics of gemcitabine and its amino acid ester prodrug following intravenous and oral administrations in mice. Biochem. Pharmacol. 2020, 180, 11412710.1016/j.bcp.2020.114127. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]
  • Dash S.; Ueda T.; Komuro A.; Honda M.; Sugisawa R.; Okada H. Deoxycytidine kinase inactivation enhances gemcitabine resistance and sensitizes mitochondrial metabolism interference in pancreatic cancer. Cell Death Dis. 2024, 15, 131.10.1038/s41419-024-06531-x. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]
  • Tang M.; Yarragudi S. B.; Pan P.; Yang K.; Kanamala M.; Wu Z. Effect of size and pH-sensitivity of liposomes on cellular uptake pathways and pharmacokinetics of encapsulated gemcitabine. J. Liposome Res. 2024, 1–11. 10.1080/08982104.2024.2389969. [Abstract] [CrossRef] [Google Scholar]
  • Crawford J.; Herndon D.; Gmitter K.; Weiss J. The impact of myelosuppression on quality of life of patients treated with chemotherapy. Future Oncol. 2024, 20, 1–16. 10.2217/fon-2023-0513. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]
  • Wang Y. Clinical effectiveness and safety of gemcitabine plus capecitabine in the treatment of advanced triple-negative breast cancer. Am. J. Transl. Res. 2024, 16, 1945–1952. 10.62347/QOWN3646. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]
  • Ciccolini J.; Serdjebi C.; Peters G. J.; Giovannetti E. Pharmacokinetics and pharmacogenetics of Gemcitabine as a mainstay in adult and pediatric oncology: an EORTC-PAMM perspective. Cancer Chemother. Pharmacol. 2016, 78, 1–12. 10.1007/s00280-016-3003-0. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]
  • Aparicio-Lopez C. B.; Timmerman S.; Lorino G.; Rogers T.; Pyle M.; Shrestha T. B.; Basel M. T. Thermosensitive Liposomes for Gemcitabine Delivery to Pancreatic Ductal Adenocarcinoma. Cancers 2024, 16, 3048.10.3390/cancers16173048. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]
  • Dora C. P.; Kushwah V.; Yadav V.; Kuche K.; Jain S. Gemcitabine-Phospholipid Complex Loaded Lipid Nanoparticles for Improving Drug Loading, Stability, and Efficacy against Pancreatic Cancer. Mol. Pharmaceutics 2024, 21, 2699–2712. 10.1021/acs.molpharmaceut.3c00983. [Abstract] [CrossRef] [Google Scholar]
  • Pereira-Silva M.; Miranda-Pastoriza D.; Diaz-Gomez L.; Sotelo E.; Paiva-Santos A. C.; Veiga F.; et al. Gemcitabine-Vitamin E Prodrug-Loaded Micelles for Pancreatic Cancer Therapy. Pharmaceutics 2024, 16, 95.10.3390/pharmaceutics16010095. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]
  • Yalcin T. E.; Ilbasmis-Tamer S.; Takka S. Antitumor activity of gemcitabine hydrochloride loaded lipid polymer hybrid nanoparticles (LPHNs): In vitro and in vivo. Int. J. Pharm. 2020, 580, 11924610.1016/j.ijpharm.2020.119246. [Abstract] [CrossRef] [Google Scholar]
  • Sercombe L.; Veerati T.; Moheimani F.; Wu S. Y.; Sood A. K.; Hua S. Advances and Challenges of Liposome Assisted Drug Delivery. Front. Pharmacol. 2015, 6, 286.10.3389/fphar.2015.00286. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]
  • Huang X.; Ding Y.; Gu J.; Tao Y.; Wu X.; Luo Q.; et al. Organ-selective lipid nanoparticles for precise cancer therapy: Beyond liposomes and polymeric micelles. Chem. Eng. J. 2024, 494, 15317110.1016/j.cej.2024.153171. [CrossRef] [Google Scholar]
  • Xu H.; Paxton J.; Lim J.; Li Y.; Zhang W.; Duxfield L.; Wu Z. Development of High-Content Gemcitabine PEGylated Liposomes and Their Cytotoxicity on Drug-Resistant Pancreatic Tumour Cells. Pharm. Res. 2014, 31, 2583–2592. 10.1007/s11095-014-1353-z. [Abstract] [CrossRef] [Google Scholar]
  • Gatto M. S.; Johnson M. P.; Najahi-Missaoui W. Targeted Liposomal Drug Delivery: Overview of the Current Applications and Challenges. Life 2024, 14, 672.10.3390/life14060672. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]
  • Mayer L. D.; Tardi P.; Louie A. C. CPX-351: a nanoscale liposomal co-formulation of daunorubicin and cytarabine with unique biodistribution and tumor cell uptake properties. Int. J. Nanosci. 2019, 14, 3819–3830. 10.2147/IJN.S139450. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]
  • Kristen M.; Plehn J.; Marchand V.; Friedland K.; Motorin Y.; Helm M.; Werner S. Manganese Ions Individually Alter the Reverse Transcription Signature of Modified Ribonucleosides. Genes 2020, 11, 950.10.3390/genes11080950. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]
  • Stiles L. I.; Ferrao K.; Mehta K. J. Role of zinc in health and disease. Clin. Experim. Med. 2024, 24, 38.10.1007/s10238-024-01302-6. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]
  • Zhukouskaya V. V.; Bardet C. Editorial: Calcium: An Overview From Physiology to Pathological Mineralization. Front. Endocrinol. 2022, 13, 93201910.3389/fendo.2022.932019. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]
  • Liang C.; Xiong N.; Liu M.; Chen Y.; Li W.; Xu J.; et al. Manganese immunotherapy for treating osteosarcoma: Glycosylating 1V209 anchored MnO2 nanosheets prompt pro-inflammatory macrophage polarization. Nano Today 2023, 48, 10167010.1016/j.nantod.2022.101670. [CrossRef] [Google Scholar]
  • Gao K.; Zhang Y.; Niu J.; Nie Z.; Liu Q.; Lv C. Zinc promotes cell apoptosis via activating the Wnt-3a/β-catenin signaling pathway in osteosarcoma. J. Orthopedic Surgery Res. 2020, 15, 57.10.1186/s13018-020-01585-x. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]
  • Anwar F.; Khan R.; Sachan R.; Kazmi I.; Rawat A.; Sabih A.; et al. Therapeutic role of calcium and vitamin K3 in chemically induced hepatocarcinogenesis – new tools for cancer treatment. Archiv. Physiol. Biochem. 2019, 125, 270–275. 10.1080/13813455.2018.1455708. [Abstract] [CrossRef] [Google Scholar]
  • Elmatbagy D.; Farahat T.; Hegazy N. N.; Barakat A.; Khattab A. The Effect of Zinc As An Adjuvant Therapy on Pneumonia in Hospitalized Children. Egyptian Family Med. J. 2024, 8, 59–75. 10.21608/efmj.2024.239863.1117. [CrossRef] [Google Scholar]
  • He J.; Zhu T.; Jiao L.; Yu L.; Peng S.; Wang Z.; et al. Surface-Engineered Polygonatum Sibiricum Polysaccharide CaCO3 Microparticles as Novel Vaccine Adjuvants to Enhance Immune Response. Mol. Pharmaceut. 2024, 21, 3936–3950. 10.1021/acs.molpharmaceut.4c00295. [Abstract] [CrossRef] [Google Scholar]
  • Qiao N.; Wang H.; Xu Y.; Chang Y.; Xie M.; Bai S.; et al. A Mn Al double adjuvant nanovaccine to induce strong humoral and cellular immune responses. J. Controlled Release 2023, 358, 190–203. 10.1016/j.jconrel.2023.04.036. [Abstract] [CrossRef] [Google Scholar]
  • Marine Chemistry: An Environmental Analytical Chemistry Approach; Gianguzza A.; Pelizzetti E.; Sammartano S., Eds.; Kluwer Academic: Dordrecht; Boston, 1997. [Google Scholar]
  • Frassineti C.; Ghelli S.; Gans P.; Sabatini A.; Moruzzi M. S.; Vacca A. Nuclear Magnetic Resonance as a Tool for Determining Protonation Constants of Natural Polyprotic Bases in Solution. Anal. Biochem. 1995, 231, 374–382. 10.1006/abio.1995.9984. [Abstract] [CrossRef] [Google Scholar]
  • Alderighi L.; Gans P.; Ienco A.; Peters D.; Sabatini A.; Vacca A. Hyperquad simulation and speciation (HySS): a utility program for the investigation of equilibria involving soluble and partially soluble species. Coord. Chem. Rev. 1999, 184, 311–318. 10.1016/S0010-8545(98)00260-4. [CrossRef] [Google Scholar]
  • Frisch M. J.et al.Gaussian 16. Revision A.03, Gaussian, Inc.: Wallingford CT, 2016. [Google Scholar]
  • Becke A. D. Density-functional exchange-energy approximation with correct asymptotic behavior. Phys. Rev. A 1988, 38, 3098–3100. 10.1103/PhysRevA.38.3098. [Abstract] [CrossRef] [Google Scholar]
  • Tomasi J.; Mennucci B.; Cammi R. Quantum Mechanical Continuum Solvation Models. Chem. Rev. 2005, 105, 2999–3094. 10.1021/cr9904009. [Abstract] [CrossRef] [Google Scholar]
  • Kühne T. D.; Iannuzzi M.; Del Ben M.; Rybkin V. V.; Seewald P.; Stein F.; et al. CP2K: An electronic structure and molecular dynamics software package - Quickstep: Efficient and accurate electronic structure calculations. J. Chem. Phys. 2020, 152, 19410310.1063/5.0007045. [Abstract] [CrossRef] [Google Scholar]
  • Goedecker S.; Teter M.; Hutter J. Separable dual-space Gaussian pseudopotentials. Phys. Rev. B 1996, 54, 1703–1710. 10.1103/PhysRevB.54.1703. [Abstract] [CrossRef] [Google Scholar]
  • VandeVondele J.; Krack M.; Mohamed F.; Parrinello M.; Chassaing T.; Hutter J. Quickstep: Fast and accurate density functional calculations using a mixed Gaussian and plane waves approach. Comput. Phys. Commun. 2005, 167, 103–128. 10.1016/j.cpc.2004.12.014. [CrossRef] [Google Scholar]
  • Krack M. Pseudopotentials for H to Kr optimized for gradient-corrected exchange-correlation functionals. Theor. Chem. Acc. 2005, 114, 145–152. 10.1007/s00214-005-0655-y. [CrossRef] [Google Scholar]
  • Perdew J. P.; Burke K.; Ernzerhof M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865–3868. 10.1103/PhysRevLett.77.3865. [Abstract] [CrossRef] [Google Scholar]
  • Grimme S.; Antony J.; Ehrlich S.; Krieg H. A consistent and accurate ab initio parametrization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu. J. Chem. Phys. 2010, 132, 15410410.1063/1.3382344. [Abstract] [CrossRef] [Google Scholar]
  • Grimme S.; Ehrlich S.; Goerigk L. Effect of the damping function in dispersion corrected density functional theory. J. Comput. Chem. 2011, 32, 1456–1465. 10.1002/jcc.21759. [Abstract] [CrossRef] [Google Scholar]
  • Bussi G.; Donadio D.; Parrinello M. Canonical sampling through velocity rescaling. J. Chem. Phys. 2007, 126, 01410110.1063/1.2408420. [Abstract] [CrossRef] [Google Scholar]
  • Xu H.; Paxton J. W.; Wu Z. Enhanced pH-Responsiveness, Cellular Trafficking, Cytotoxicity and Long-circulation of PEGylated Liposomes with Post-insertion Technique Using Gemcitabine as a Model Drug. Pharm. Res. 2015, 32, 2428–2438. 10.1007/s11095-015-1635-0. [Abstract] [CrossRef] [Google Scholar]
  • Filella M.; May P. Reflections on the calculation and publication of potentiometrically-determined formation constants. Talanta 2005, 65, 1221–1225. 10.1016/j.talanta.2004.08.046. [Abstract] [CrossRef] [Google Scholar]
  • Carnamucio F.; Foti C.; Cordaro M.; Giuffrè O. Study on Metronidazole Acid-Base Behavior and Speciation with Ca2+ for Potential Applications in Natural Waters. Molecules 2022, 27, 5394.10.3390/molecules27175394. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]
  • Aiello D.; Carnamucio F.; Cordaro M.; Foti C.; Napoli A.; Giuffrè O. Ca2+ Complexation With Relevant Bioligands in Aqueous Solution: A Speciation Study With Implications for Biological Fluids. Front. Chem. 2021, 9, 64021910.3389/fchem.2021.640219. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]
  • Reddy P. R.; Rao V. B. M. Role of secondary ligands in the structure and stability of metal—cytidine complexes in solution. Polyhedron 1985, 4, 1603–1609. 10.1016/S0277-5387(00)87235-6. [CrossRef] [Google Scholar]
  • Smith R. M.; Martell A. E.; Chen Y. Critical Evaluation of stability constants for nucleotide complexes with protons and metal ions and the accompanying enthalpy changes. Pure Appl. Chem. 1991, 63, 1015–1080. 10.1351/pac199163071015. [CrossRef] [Google Scholar]
  • Carnamucio F.; Foti C.; Micale N.; Van Pelt N.; Matheeussen A.; Caljon G.; Giuffrè O. Metronidazole Interaction with Cu2+ and Zn2+: Speciation Study in Aqueous Solution and Biological Activity Evaluation. ACS Omega 2024, 9, 29000–29008. 10.1021/acsomega.4c04166. [Europe PMC free article] [Abstract] [CrossRef] [Google Scholar]
  • Rezkallah E.; Ibrahim A.; Dahy A.; Hakiem A. A.; Mahfouz R. DFT and Thermal Decomposition Studies on Gemcitabine. Z. Phys. Chem. 2019, 233, 1503–1527. 10.1515/zpch-2018-1304. [CrossRef] [Google Scholar]
  • Najafi F. Thermodynamic studies of carbon nanotube interaction with Gemcitabine anticancer drug: DFT calculations. J. Nanostruct. Chem. 2020, 10, 227–242. 10.1007/s40097-020-00344-y. [CrossRef] [Google Scholar]
  • Rudolph W. W.; Irmer G. Hydration and speciation studies of Mn2+ in aqueous solution with simple monovalent anions (ClO4–, NO3–, Cl–, Br–). Dalton Trans. 2013, 42, 14460.10.1039/c3dt51493e. [Abstract] [CrossRef] [Google Scholar]

Full text links 

Read article at publisher's site: https://doi.org/10.1021/acsami.4c12550

Similar Articles 

To arrive at the top five similar articles we use a word-weighted algorithm to compare words from the Title and Abstract of each citation.

Funding 

Funders who supported this work.

European Commission